Sketch
Sector Admin

Posts: 2,939 | Re: MAGI's CSG Primitives?
on Friday, August, 04, 2006 11:21 AM
July 1982 is the issue.
This is the cover http://www.tron-sector.com/gallery/show.aspx?id=1142
This is the text block that goes with the above outline cycle:
"At MAGI, cycle is created out of 57 geometric forms (Some outlined in red), combined in computer."
The articles are very informative. The part where they discuss MAGI you all might be interested in reading.
If your really wanting info, might also check the August 1982 issue of American Cinematographer, which has lenghty articles about the film.
I could scan some of the arcitles, when there's time, but would rather email them to one of you, for posting them would take way too long.
I would look into getting these issues off ebay if I where you, they are worth getting.
https://www.flickr.com/photos/blue_bezel/
|
wwwmwww
User

Posts: 1,231 | Re: MAGI's CSG Primitives?
on Friday, August, 04, 2006 11:41 AM
| Sketch Wrote:I could scan some of the arcitles, when there's time, but would rather email them to one of you, for posting them would take way too long. |
I'll be happy to post them if you emailed them to me.
| Sketch Wrote:I would look into getting these issues off ebay if I where you, they are worth getting. |
I'll do that too.
Thanks,
Carl

|
matthew180
User

Posts: 114 | Re: MAGI's CSG Primitives?
on Friday, August, 04, 2006 7:53 PM
If you want to email them to me also, I'll make sure they get on a fast server for anyone else who may be interested. Some high-resolution scans are all that would be necessary.
Carl, if you are going to bid on ebay, let me know so we don't compete with each other. I'm looking now and have only found July 1982 in a "bound volume", whatever that is, and in a 125 magazine collection!
I also found some interesting info about Synthavision on a page written by Ken Perlin, he says this:
"One of the factors that influenced me was the fact that MAGI's SynthaVision system did not use polygons. Rather, everything was built from boolean combinations of mathematical primitives, such as ellipsoids, cylinders, truncated cones. As you can see in the illustration, the lightcycles are created by adding and subtracting simple solid mathematical shapes."
Here is the link, look at chaper 2:
http://www.csee.umbc.edu/~olano/s2001c24/
That's interesting because I combined the three parts of the sides into a single polygon, shown below, but they would not have been able to do it that way. Looks like they would have had to use 2 rectangles and 3 triangles, which are also seen on the DVD construction video. I sure wish he listed more of the polygons.

Also, from that awesome new image posted by Protos, it looks like the canopy taper is really a torus... However it could also be a cut cone, which is what I used.
Matthew
How are you going to control the universe if you can't answer a few unsolvable problems?
|
wwwmwww
User

Posts: 1,231 | Re: MAGI's CSG Primitives?
on Saturday, August, 05, 2006 3:04 PM
| matthew180 Wrote:Carl, if you are going to bid on ebay, let me know so we don't compete with each other. I'm looking now and have only found July 1982 in a "bound volume", whatever that is, and in a 125 magazine collection! |
Feel free. I'll let you have first dibs. Here is that Art of TRON book I mentioned via email:
http://www.tron-sector.com/gallery/show.aspx?ID=37
matthew180 Wrote:I also found some interesting info about Synthavision on a page written by Ken Perlin, he says this:
"One of the factors that influenced me was the fact that MAGI's SynthaVision system did not use polygons. Rather, everything was built from boolean combinations of mathematical primitives, such as ellipsoids, cylinders, truncated cones. As you can see in the illustration, the lightcycles are created by adding and subtracting simple solid mathematical shapes."
Here is the link, look at chaper 2:
http://www.csee.umbc.edu/~olano/s2001c24/
That's interesting because I combined the three parts of the sides into a single polygon, shown below, but they would not have been able to do it that way. Looks like they would have had to use 2 rectangles and 3 triangles, which are also seen on the DVD construction video. I sure wish he listed more of the polygons. |
Well by rectangles I assume you mean boxes and by triangles I assume you mean triangular prisims. If so I don't recall seeing 5 primitives used to make the shape you describe. As I recall what is seen on the DVD is 1 triangular prisim and 2 right trapezoidal prisims. However if MAGI's software could extrude a right trapezoid into a prisim I really don't see why it could extrude any polygon into a prism.
| matthew180 Wrote:Also, from that awesome new image posted by Protos, it looks like the canopy taper is really a torus... However it could also be a cut cone, which is what I used. |
I don't see it being a torus but it could be an elipsoid which is what I've just changed to. It saved me another 2 primitives and after looking at several screen shots and other images in this thread I'm now convinced I see some curvature to the sides of the canopy. And while we are talking about that image look at this:

The one I have the top red arrow pointing to I now believe to be the window cutter. Much as you used here:

I think this can also be seen on DVD construction video to be the case. Personally I don't see why they didn't use the same cutter here as was used for the wings. That's what I did.
Below that cutter I see two large right trapezoidal prisim cutters. One for the wings and one for the top part of the side. However I noticed there is another box or prisim sticking out just above these indicated by the blue arrows. What would this be for? And I still don't have a clue about the 3 odd lines seen behind the light cycle.
I wonder if one of them might be related to the rear wheel cutter. At the moment my model uses 2 spheres but I'm starting to wonder if that cutter should really be just a strait cylinder. I now need to go back and see if I can convince myself that there is or isn't a taper in from the edge of he rear wheel cut to the start of the rear wheel hub. If you have any good shots of this area please post. Maybe I can get rid of one more primitive.
Carl

|
wwwmwww
User

Posts: 1,231 | Re: MAGI's CSG Primitives?
on Saturday, August, 05, 2006 3:36 PM
Here is where my new geometry stands at the moment:
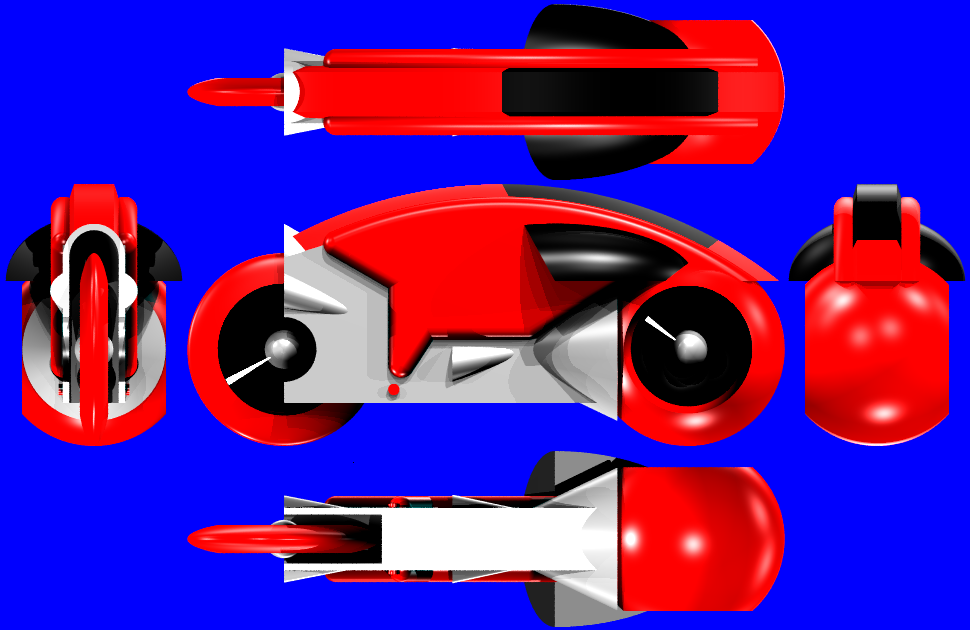
Comments?
Carlwhere to buy abortion pill http://blog.bitimpulse.com/template/default.aspx?abortion-types buy abortion pill online

|
matthew180
User

Posts: 114 | Re: MAGI's CSG Primitives?
on Sunday, August, 06, 2006 4:31 PM
| wwwmwww Wrote:Well by rectangles I assume you mean boxes and by triangles I assume you mean triangular prisims. If so I don't recall seeing 5 primitives used to make the shape you describe. As I recall what is seen on the DVD is 1 triangular prisim and 2 right trapezoidal prisims. However if MAGI's software could extrude a right trapezoid into a prisim I really don't see why it could extrude any polygon into a prism. |
Correct, rectangle == cubes, boxes, etc. and triangles == prisms, etc.. I guess, technically, a polygon in 3D is called a polyhedron. I don't know if they could to trapezoids. They may have had to use a sheer transformation on a cube to get the shape instead of just having the primitive directly? But Perlin started right there that they could not use polygons, so I have to assume the available primitive set was pretty limited, thus a lot of boolean operations to get some shapes we can do directly, like this:

| wwwmwww Wrote:I don't see it being a torus but it could be an elipsoid which is what I've just changed to. It saved me another 2 primitives and after looking at several screen shots and other images in this thread I'm now convinced I see some curvature to the sides of the canopy. |
Do you have any screenshots that show this clearly? It would save primitives, but we do know they had cut-cones which could do the same thing, albeit with 1 more primitive. It seems it would be a pain in the butt to get an ellipse into the exact shape needed. The wing ellipse was probably the hardest shape for me to get just right.
| wwwmwww Wrote:And while we are talking about that image look at this: |
I think that big part sticking out the top is the windshield cutter and/or the part that makes the windshield black.
The part you are pointing to with blue arrows looks like a box used to AND with the canopy (just the canopy cylinder and taper), and the predominantly larger box seen here is used to AND with the canopy shoulders and upper half of the sides.
The large circular shape (rooster tail looking), could be the jet wall arc or the wheel well cutter. I originally cut my wheel well with another torus, but later changed it to a sheered cylinder.
The two smaller parts in the back, I have no idea. It's too hard to determine from this angle. What I want to know is, what is that circle on the front wheel?

| wwwmwww Wrote:I wonder if one of them might be related to the rear wheel cutter. At the moment my model uses 2 spheres but I'm starting to wonder if that cutter should really be just a strait cylinder. I now need to go back and see if I can convince myself that there is or isn't a taper in from the edge of he rear wheel cut to the start of the rear wheel hub. If you have any good shots of this area please post. Maybe I can get rid of one more primitive. |
I always thought the inside of the rear wheel was tapered in a little too, but I'll have to go back and look at some images now. I'm currently using two ellipses as cutters as well. I guess it would make more sense to be cut with a cylinder since the rear hub is a cylinder (a very thin cylinder.)
I have to run right now, I'll comment on your all-view image in a bit, but it's looking really good!
Matthew
How are you going to control the universe if you can't answer a few unsolvable problems?
|
wwwmwww
User

Posts: 1,231 | Re: MAGI's CSG Primitives?
on Sunday, August, 06, 2006 7:43 PM
| matthew180 Wrote:Correct, rectangle == cubes, boxes, etc. and triangles == prisms, etc.. I guess, technically, a polygon in 3D is called a polyhedron. |
Well a polydedron is a much more general classification of 3D shapes then what we are talking about. We are talking about the subset of polyhedrons which are prisms, which amount to a polydon extruded in a third dimension. A box for example is a subset of the prisms as its a rectangle extruded in a third dimention.
| matthew180 Wrote:I don't know if they could to trapezoids. They may have had to use a sheer transformation on a cube to get the shape instead of just having the primitive directly? |
Well you can't sheer a cube into a right trapezoidal prism. If you sheer one side you sheer the otherside as well.
| matthew180 Wrote:But Perlin started right there that they could not use polygons, so I have to assume the available primitive set was pretty limited, thus a lot of boolean operations to get some shapes we can do directly, like this: |
Maybe, but I took Perlin's statement to mean the model wasn't a mesh made up of polygons, simple 2D shapes. I think he's simply saying it is a CSG made up of sold 3D shapes which have volume. Keep in mind the sides of a box are polygons and we agree a box is an allowed primitive. So yes they must have been able to "use" polygons. Its just that as with a box you model with the whole volume and not just the polygons that make up the surface.
matthew180 Wrote o you have any screenshots that show this clearly? o you have any screenshots that show this clearly? |
Using this:
http://www.tron-sector.com/gallery/show.aspx?ID=2327
I made this:
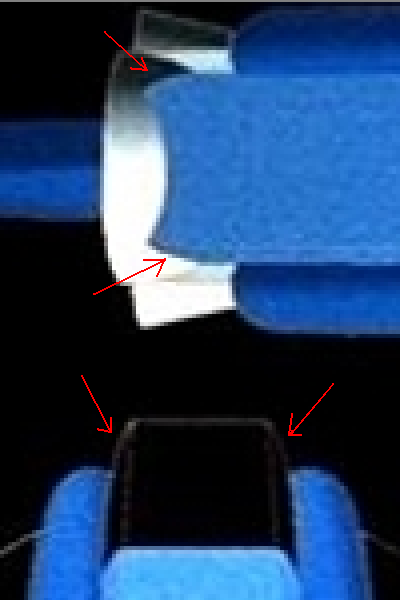
From the front view I believe the sides indicated by the arrows are curved. So both of these surfaces could be part of one big ellipsoid.
Looking from the top where this surface hits the fender again it looks like this surface is curved outward. If these surfaces were made from two cones and they intersected a cylinder in this fashion the line of intersection would actually be curved inward (not outward).
| matthew180 Wrote:It would save primitives, but we do know they had cut-cones which could do the same thing, albeit with 1 more primitive. It seems it would be a pain in the butt to get an ellipse into the exact shape needed. |
Well you currently have two cones defined by a center and a radius each... correct? If so these two circles uniquely define an elliposoid. The center of the elliposid should be trivial to determine. Now you just need the radius and the scaling factor. In my case the two circles are in different z-planes call them z1 and z2. Say the circle in the z1 plane has a radius of r1 and the circle in the z2 plane has a radius of r2. You know the center of the ellipsoid is at x0,y0,z0. So you have these equations:
(x-x0)^2+(y-y0)^2+(z-z0)^2=r^2
r1^2+(s*z1)^2=r^2
r2^2+(s*z2)^2=r^2
Just solve for s (your scale factor) and r (your radius).
And this is just me being picky but an ellipse is a 2D shape, an ellipsoid is a 3D shape.
| matthew180 Wrote:I think that big part sticking out the top is the windshield cutter and/or the part that makes the windshield black. |
Agreed.
| matthew180 Wrote:The part you are pointing to with blue arrows looks like a box used to AND with the canopy (just the canopy cylinder and taper), and the predominantly larger box seen here is used to AND with the canopy shoulders and upper half of the sides. |
Ok... I can see that.
| matthew180 Wrote:The large circular shape (rooster tail looking), could be the jet wall arc or the wheel well cutter. I originally cut my wheel well with another torus, but later changed it to a sheered cylinder. |
Torus... really? Or do you mean ellipsoid? And I can't<

|
matthew180
User

Posts: 114 | Re: MAGI's CSG Primitives?
on Monday, August, 07, 2006 1:18 AM
| wwwmwww Wrote:Well a polydedron is a much more general classification of 3D shapes then what we are talking about. We are talking about the subset of polyhedrons which are prisms, which amount to a polydon extruded in a third dimension. A box for example is a subset of the prisms as its a rectangle extruded in a third dimention. |
I think this covers pretty much what we are talking about. Your use of the term "prism" seems to be technically accurate, I ignorantly applied the term generally to triangular shapes.
http://en.wikipedia.org/wiki/Prism_%28geometry%29
| wwwmwww Wrote:Maybe, but I took Perlin's statement to mean the model wasn't a mesh made up of polygons, simple 2D shapes. I think he's simply saying it is a CSG made up of sold 3D shapes which have volume. Keep in mind the sides of a box are polygons and we agree a box is an allowed primitive. So yes they must have been able to "use" polygons. Its just that as with a box you model with the whole volume and not just the polygons that make up the surface. |
I'm not sure about this. In a mesh-based 3D program you could argue that all shapes end up as triangular polygons. However, with primitives, the software does not evaluate the surface as, say, polygonal triangles. Instead, a circle primitive is represented with a center point and radius and the surface is calculated to whatever precision necessary and not that of the density of triangles making up a mesh surface.
When Perlin said they could not do polygons ( http://en.wikipedia.org/wiki/Polygon), I take it to mean they could not create arbitrary shapes from a defined set of points in 2D, and then have the polygon extruded to some depth to make a 3D object (prism). Similar to the way you work with primitives in POV, I think the software knew of a limited set of primitive objects to which you could provide the various parameters, xyz center point and radius for a sphere, xyx and length width height for a cuboid, xyz center point, radius1 and radius2 for a torus, etc., then the ability to perform boolean operations on those objects, i.e. CSG (check out the example image for the Wikipedia page for CSG):
http://en.wikipedia.org/wiki/Constructive_solid_geometry
wwwmwww Wrote:From the front view I believe the sides indicated by the arrows are curved. So both of these surfaces could be part of one big ellipsoid.
Looking from the top where this surface hits the fender again it looks like this surface is curved outward. If these surfaces were made from two cones and they intersected a cylinder in this fashion the line of intersection would actually be curved inward (not outward). |
Actually, it looks like they make a straight line:

You make a good argument though, I'd like to see a close up of this area once you swap out the cones for ellipsoids.
wwwmwww Wrote:Well you currently have two cones defined by a center and a radius each... correct? If so these two circles uniquely define an elliposoid. The center of the elliposid should be trivial to determine. Now you just need the radius and the scaling factor. In my case the two circles are in different z-planes call them z1 and z2. Say the circle in the z1 plane has a radius of r1 and the circle in the z2 plane has a radius of r2. You know the center of the ellipsoid is at x0,y0,z0. So you have these equations:
(x-x0)^2+(y-y0)^2+(z-z0)^2=r^2
r1^2+(s*z1)^2=r^2
r2^2+(s*z2)^2=r^2
Just solve for s (your scale factor) and r (your radius). |
I'm not sure I follow what your trying to determine here. I think you are describing how to determine a single ellipsoid to replace the two cones I'm currently using, based on the size and placement of those existing cones? But I'm not quite following this.
| wwwmwww Wrote:where to buy abortion pill abortion types buy abortion pill online |
How are you going to control the universe if you can't answer a few unsolvable problems?
|
wwwmwww
User

Posts: 1,231 | Re: MAGI's CSG Primitives?
on Monday, August, 07, 2006 12:24 PM
| matthew180 Wrote:Your use of the term "prism" seems to be technically accurate, I ignorantly applied the term generally to triangular shapes. |
No biggie. A triangular prisim is the one most people are introduced to first as that is what is typically used in science classes to refract light.
| matthew180 Wrote:I'm not sure about this. In a mesh-based 3D program you could argue that all shapes end up as triangular polygons. |
All polygons can be cut up into triangles. I don't think the term triangular polygon means much unless it simply means triangles.
| matthew180 Wrote:However, with primitives, the software does not evaluate the surface as, say, polygonal triangles. Instead, a circle primitive is represented with a center point and radius and the surface is calculated to whatever precision necessary and not that of the density of triangles making up a mesh surface. |
Agreed.
| matthew180 Wrote:When Perlin said they could not do polygons (http://en.wikipedia.org/wiki/Polygon), I take it to mean they could not create arbitrary shapes from a defined set of points in 2D, and then have the polygon extruded to some depth to make a 3D object (prism). Similar to the way you work with primitives in POV, I think the software knew of a limited set of primitive objects to which you could provide the various parameters, xyz center point and radius for a sphere, xyx and length width height for a cuboid, xyz center point, radius1 and radius2 for a torus, etc., then the ability to perform boolean operations on those objects, i.e. CSG |
Well you believe MAGI could make a triangular prisim... correct? How do you do that without defining some points in 2D? I guess if you have no prism primitive you could make a triangular prism by cutting one box with another box. In that case your shape above that you made with 5 primitives could be made with 8 boxes. At the end of the day I have to say I don't know just what primitives MAGI had to play with but if I can get the same end result with fewer POV-Ray primitives that is what I'm going to do.
| matthew180 Wrote:Actually, it looks like they make a straight line: |
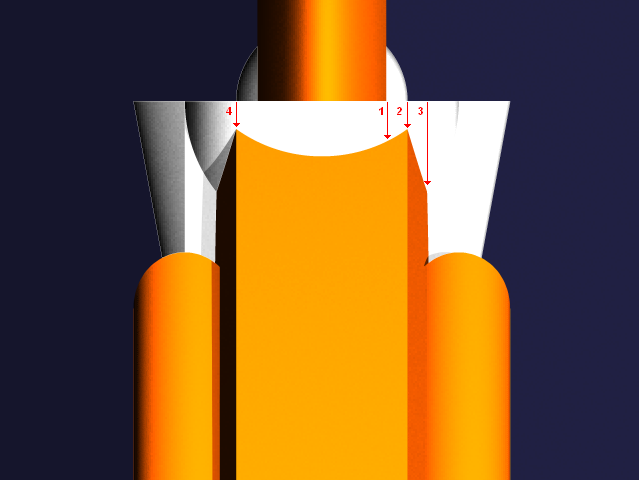
I think its a matter of scale. Look at the line of intersection between arrow 4 and 2. This is curved inwards however can you tell that if you just examine the portion of that curve between arrows 1 and 2? Arrows 1 and 2 have the same horizontal displacement as arrows 2 and 3 and I think they should contain the same amouth of inward curvature.
| matthew180 Wrote:You make a good argument though, I'd like to see a close up of this area once you swap out the cones for ellipsoids. |
Here you go...
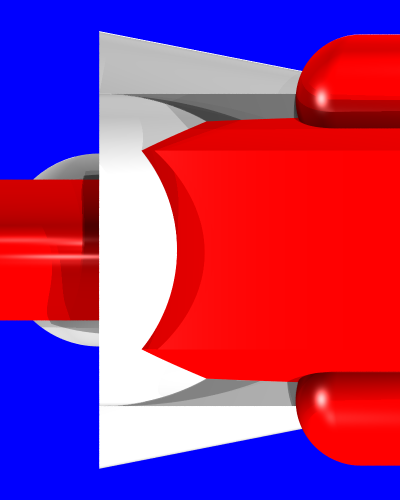
| matthew180 Wrote:I'm not sure I follow what your trying to determine here. I think you are describing how to determine a single ellipsoid to replace the two cones I'm currently using, based on the size and placement of those existing cones? But I'm not quite following this. |
Its just some algebra... you have two equations and two unknowns. I unlike most people like math and I love working out geometry problems like this. If you send me your two center points and the two radai used to define your cones I'll calculate the ellipsoid center, radai, and scalling factor for you.
| matthew180 Wrote:I'm pretty sure I got the wheel well correct. Look at these, the one on the left is from the all-view image, the one on the right is a screen capture from the DVD: |
Agreed... the wheel well is correct. I for a moment there thought we were talking about the shape that cuts the rear wheel itself.
| matthew180 Wrote:You would not get those circular outlines if you used a cylinder to cut th |

|
wwwmwww
User

Posts: 1,231 | Re: MAGI's CSG Primitives?
on Monday, August, 07, 2006 12:31 PM
Here you go...

I found that in this thread... which I'm not sure if I'm pointed you to before or not. If not its another great thread for you to check out.
http://www.tron-sector.com/forums/default.aspx?do=top&id=331276
Carl

|
matthew180
User

Posts: 114 | Re: MAGI's CSG Primitives?
on Tuesday, August, 08, 2006 12:43 PM
| wwwmwww Wrote:Well you believe MAGI could make a triangular prisim... correct? How do you do that without defining some points in 2D? I guess if you have no prism primitive you could make a triangular prism by cutting one box with another box. In that case your shape above that you made with 5 primitives could be made with 8 boxes. At the end of the day I have to say I don't know just what primitives MAGI had to play with but if I can get the same end result with fewer POV-Ray primitives that is what I'm going to do. |
I think Perlin was just using Synthavision and bound by what was available. It only makes sense that, somehow, they could describe a 2D polygon (box, triangle, etc.) outline and extend it in the Z direction to make a prism, but I think those were hard-wired in the code and there was no facility to allow the user to specify their own 2D polygons for extrusion. Just a hunch really, until the day someone who worked directly with Synthavision comes forward to tell us for sure.
I take your stance as well, I'm already using prisms in various places such as the chassis and sides.
matthew180 Wrote:Actually, it looks like they make a straight line:
| wwwmwww Wrote:I think its a matter of scale. Look at the line of intersection between arrow 4 and 2. This is curved inwards however can you tell that if you just examine the portion of that curve between arrows 1 and 2? Arrows 1 and 2 have the same horizontal displacement as arrows 2 and 3 and I think they should contain the same amount of inward curvature. |
|
I thought you were looking for outward curvature where the canopy-taper intersects the fender? Isn't that what you were pointing to here?

| wwwmwww Wrote:Its just some algebra... you have two equations and two unknowns. I unlike most people like math and I love working out geometry problems like this. If you send me your two center points and the two radai used to define your cones I'll calculate the ellipsoid center, radai, and scalling factor for you. |
The radius is 120 units. X1,2 and Y1,2 are the same, Z1 and Z2 are 10 units apart.
wwwmwww Wrote:Agreed... the wheel well is correct. I for a moment there thought we were talking about the shape that cuts the rear wheel itself.
...snip...
But you used a cylinder here didn't you? I used a sheered cylinder in this area.
...snip...
I'm lost. If you aren't using a cylinder what shape are you using? |
I think we got out of sync on this somehow. I was talking about the mystery cylinders in that new superimposed wire-frame image Protos posted (we really need a name for that image), and you were thinking about real-wheel cutters, and it all went down hill from there. I do use a sheered cylinder for the fender and fender/wheel well cutter, as well as a cuboid and additional cylinder (as seen in the assembly image in my last post.)
wwwmwww Wrote:| matthew180 Wrote:If that is true, then we now know exactly how thick the "rim" is on the front wheel. What I'm calling the "rim" is the inside part of the front wheel that is the wheel color before turning black. |
Maybe, after playing with that assumption a bit I'm now not sure. I think that makes the rim too deep on my model to look right. It may be something else. |
I thought it might be too deep, but I was going to try it before saying so. Now we know, it's too deep going on your initial thought. I'd still like to know what that circle is though.
| wwwmwww Wrote:GREAT pic!!! Thanks. |
Sure thing. The explosion frames can be great places to see individual parts.
| wwwmwww Wrote:You are operating on the assumption that these parts don't stick out past the body... correct? You may be right but I'm not sure. Iforder abortion pill http://unclejohnsprojects.com/template/default.aspx?morning-after-pill-price where to buy abortion pill |
How are you going to control the universe if you can't answer a few unsolvable problems?
|
wwwmwww
User

Posts: 1,231 | Re: MAGI's CSG Primitives?
on Thursday, August, 10, 2006 10:30 AM
| matthew180 Wrote:I think Perlin was just using Synthavision and bound by what was available. It only makes sense that, somehow, they could describe a 2D polygon (box, triangle, etc.) outline and extend it in the Z direction to make a prism, but I think those were hard-wired in the code and there was no facility to allow the user to specify their own 2D polygons for extrusion. Just a hunch really, until the day someone who worked directly with Synthavision comes forward to tell us for sure. |
Its certainly a possibility.
| matthew180 Wrote:I thought you were looking for outward curvature where the canopy-taper intersects the fender? Isn't that what you were pointing to here? |
Correct. That is what I'm trying to copy. I believe my attempt above is still too strait though I think it may have a very small amout of outward curvature. Where I referenced to curve where the canopy top intersects the fender it was just for comparision. I was saying if you only look at the same horizontal sized segment of this curve you can't really tell its curved either though when you see the whole thing its obviously curved.
| matthew180 Wrote:The radius is 120 units. X1,2 and Y1,2 are the same, Z1 and Z2 are 10 units apart. |
A cone has two radii. I only see one radius above. Also are your two z's above from the same cone or from cones on opposite sides of the bike as I suspect? If so I still need another z value and a radius.
| matthew180 Wrote:I think we got out of sync on this somehow. I was talking about the mystery cylinders in that new superimposed wire-frame image Protos posted (we really need a name for that image), and you were thinking about real-wheel cutters, and it all went down hill from there. I do use a sheered cylinder for the fender and fender/wheel well cutter, as well as a cuboid and additional cylinder (as seen in the assembly image in my last post.) |
Ok... I understand now.
| matthew180 Wrote:I thought it might be too deep, but I was going to try it before saying so. Now we know, it's too deep going on your initial thought. I'd still like to know what that circle is though. |
Don't let my thoughts stop you from trying it. I may have some other dimensions far enough off that its keeping it from looking correct. The circle appears to be in the same plane as the side panel. Why the intersection of this plane and the front wheel would have a line on it like that though I'm not sure. Maybe my side panels need to be pulled out a bit.
| matthew180 Wrote:Also, I've been messing with using a single ellipsoid for the canopy taper, but I cannot reproduce the outward curve where the taper intersects the fender. The best I get is a straight line similar to what using cones produced. |
I'm not happy with the amout of curvature I'm getting either. I still need to play with this shape. One thought that did cross my mind is maybe its not an ellipsoid. A torus could be cut up in a similiar fashion and if done that way it might not have to be scaled as much so maybe more curvature could be maintained. I'll try that but I still have more tinkering I need to do before I give up on the ellipsoid and this week has been crazy at work so I've not done much since my last post. There is already talk of working though the weekend so be patient as I may not have much time to play with this for the next several days.
Carl
where to buy abortion pill http://blog.bitimpulse.com/template/default.aspx?abortion-types buy abortion pill online

|
matthew180
User

Posts: 114 | Re: MAGI's CSG Primitives?
on Friday, August, 11, 2006 11:45 PM
| wwwmwww Wrote:A cone has two radii. I only see one radius above. Also are your two z's above from the same cone or from cones on opposite sides of the bike as I suspect? If so I still need another z value and a radius. |
Well, my cones have a circular base and a point, so if there are two radii, they are the same. Here is an image:

| wwwmwww Wrote:I'm not happy with the amout of curvature I'm getting either. I still need to play with this shape. One thought that did cross my mind is maybe its not an ellipsoid. A torus could be cut up in a similiar fashion and if done that way it might not have to be scaled as much so maybe more curvature could be maintained. I'll try that but I still have more tinkering I need to do before I give up on the ellipsoid and this week has been crazy at work so I've not done much since my last post. There is already talk of working though the weekend so be patient as I may not have much time to play with this for the next several days. |
Take as much time as you need to. I have a little job searching to do myself. In the meantime, I received my copy of the August 1982 American Cinematographer, which is very cool by the way! A huge part of the magazine is about TRON and I'll be getting some of the info into digital form. In the mean time, I just could not wait to post these images. They are only about 2" x 2" in the magazine, so I scanned them at 600 dpi and zoomed in until I could see the screen used to print them (if anyone wants my 6.5MB 600dpi scanned image, let me know). The caption reads:
"A sampler of the basic geometric shapes used in the construction of data bases for objects in the MAGI computer."


How about that!!  Anyone who has this magazine issue, you have been holding out on us!! All bets are off now as to what MAGI could and could not do. Some of those shapes go well beyond what I would call "basic". I realize they are not all "primitives" and we are seeing some CSG here, but still. Anyway, I had to share those. If we are going to reference objects in these images, just go sequentially left to right, top to bottom. Also note that the "look" of TRON that we were talking about a while back, was not due to lack of technology or a loss of data during transfer to film (which they could all do directly), again from the article:
"Taylor and director Steven Lisberger had selected these companies from the handful of computer graphics companies capable of meeting the standards required for images to be projected on a theater screen. They all work with systems having at least 2000 lines of resolution. Triple I has a film recorder capable of 4,000 horizontal lines with 6,000 pixels per line, which Taylor estimates exceeds the resolving power of 5247 color negative by 60%. In addition the companies doing computer simulation for TRON had to have programs which were sophisticated enough to eliminate aliasing and rastering (defects often occurring in computer generated images which give objects jagged edges and make them look artificial), and they needed to be able to achieve a variety of other effects which enhance the realism of the images such as "depth clueing" which alters the brightness of an object according to how far away it is from the theoretical camera view point."
Very cool information! More to come I'm sure.
Matthew
order abortion pill http://unclejohnsprojects.com/template/default.aspx?morning-after-pill-price where to buy abortion pill
How are you going to control the universe if you can't answer a few unsolvable problems?
|
wwwmwww
User

Posts: 1,231 | Re: MAGI's CSG Primitives?
on Saturday, August, 12, 2006 6:23 PM
| matthew180 Wrote:Well, my cones have a circular base and a point, so if there are two radii, they are the same. |
Ok... I see the issue now. In POV-Ray a cone is defined in this fassion:
cone {
, r1 // Center and radius of one end
, r2 // Center and radius of other end
}
Your second radius is zero as you allow your cone to come to a point. I used a cone that was cut off very near the base. This gives me two set of circles on each side of the light cycle. Given this set of circles I can fit two cones to them or if I like I can simply find 1 ellipsoid that passes through all 4 circles. Or if I want to get really fancy I can also find a scaled torus that has a surface that contains all 4 circles as well.
Check out this example:

The surface of the torus is the most curved so maybe that's the correct shape to use.
Carl

|
wwwmwww
User

Posts: 1,231 | Re: MAGI's CSG Primitives?
on Saturday, August, 12, 2006 6:46 PM
matthew180 Wrote:
"A sampler of the basic geometric shapes used in the construction of data bases for objects in the MAGI computer."
|
Well the first object in the second row looks like what POV-Ray would call a Lathe Object. That is if it isn't two cones with a torus subtracted from them. The Lathe Object could be considered one primitive. If its the later its a CSG made of 3 primitives.
Also the last object in the second row looks to be what POV-Ray would call a linear_sweep cubic_spline prism defined by extruding a curved shape defined by over 5 points into the 3rd dimention. If MAGI could do this they certainly could make the linear_sweep linear_spline prisims which you've been wanting to break up into boxes and triangular prisms.
Carl

|
matthew180
User

Posts: 114 | Re: MAGI's CSG Primitives?
on Saturday, August, 12, 2006 10:43 PM
| wwwmwww Wrote:Also the last object in the second row looks to be what POV-Ray would call a linear_sweep cubic_spline prism defined by extruding a curved shape defined by over 5 points into the 3rd dimention. If MAGI could do this they certainly could make the linear_sweep linear_spline prisims which you've been wanting to break up into boxes and triangular prisms. |
Absolutely! But every image we see, i.e. the construction sequence on the DVD, and the image with view-frame overlay, show those side pieces as three or so primitives... I think they could do it with one, as we have just seen.
Also, what about the third shape in the first row? Look at that surface, the curves are pretty complex and is either a mesh of some sort, or a lot of subtractions. Anyway, I found the images very enlightening.
As for the canopy taper, I think I'll try a torus as you have shown and see what kind of intersection I get with the fender. Thanks for the graphic.
Matthew
where to buy abortion pill http://blog.bitimpulse.com/template/default.aspx?abortion-types buy abortion pill online
How are you going to control the universe if you can't answer a few unsolvable problems?
|
wwwmwww
User

Posts: 1,231 | Re: MAGI's CSG Primitives?
on Sunday, August, 13, 2006 9:07 AM
| matthew180 Wrote:Absolutely! But every image we see, i.e. the construction sequence on the DVD, and the image with view-frame overlay, show those side pieces as three or so primitives... I think they could do it with one, as we have just seen. |
I agree. Maybe the reason they broke it up was to give them more pieces to play with for the crash effect. Maybe they wanted to restrict themselves to a simple subset of the primitives available to them just to aid in the computer generated look of the light cycle. It's also possible rendering times played a factor in their selection of primitives to use.
| matthew180 Wrote:Also, what about the third shape in the first row? Look at that surface, the curves are pretty complex and is either a mesh of some sort, or a lot of subtractions. Anyway, I found the images very enlightening. |
I think its either what POV-Ray would call a Isosurface Object or something similiar to what POV-Ray calls a Bicubic Patch Object.
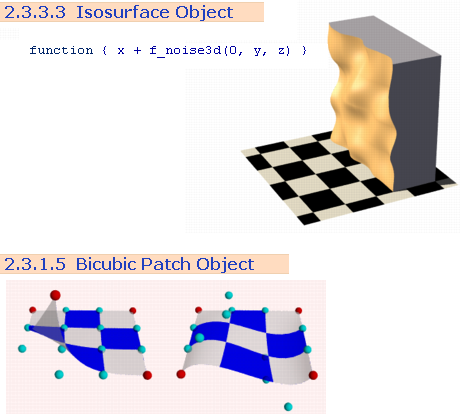
I'm leaning toward the Isosurface as I tend to think of the Bicubic Patch as a type of 2D mesh. However the lines on the shape make me think of the control points used to define a Bicubic Patch so maybe they could define a function with the use of control points in their Isosurface generator.
Check out the torus like shape in the second row. It also has the extra lines and it appears the hole in the center has a square cross-section. If that's the case it almost has to be an Isosurface.
| matthew180 Wrote:As for the canopy taper, I think I'll try a torus as you have shown and see what kind of intersection I get with the fender. Thanks for the graphic. |
I'll do the same and see what I get. I was about 99% of the way there yesterday but just ran out of time.
Carl

|
wwwmwww
User

Posts: 1,231 | Re: MAGI's CSG Primitives?
on Monday, August, 14, 2006 4:14 PM
Here is a closeup of the fender with the 3 construction types used. Look at the upper part of the image. Something odd happened on the bottom half as I was making the gif. Not sure what exactly, but it does help show the lines of intersection for the 3 different shapes.
And I should also point out that if there appears to be too much curvature for this torus shape, this torus isn't the only one that has a surface containing the 4 circles mentioned above. If fact you can think of the ellipsoid as a torus with a major radius of zero and the major radius should be able to be continously varied up to the value used in this torus as long as the shape is scaled appropriately and the proper minor radius is used.
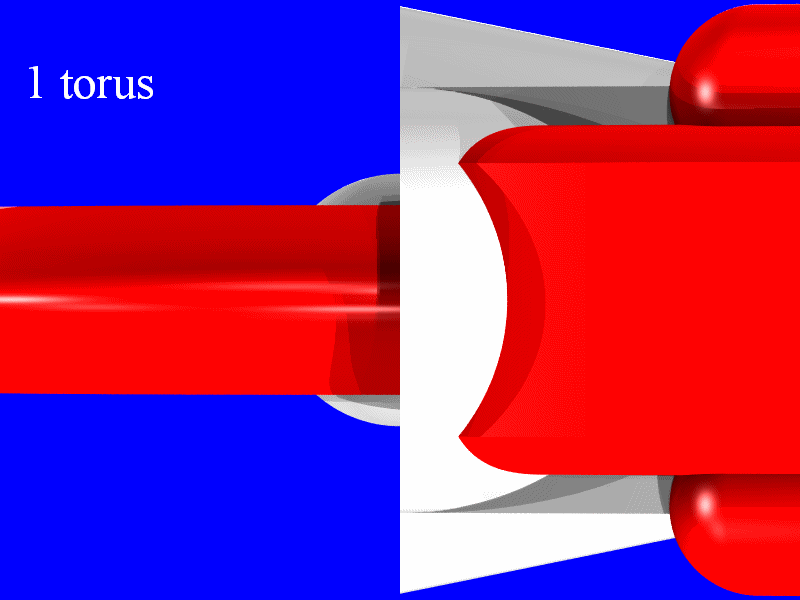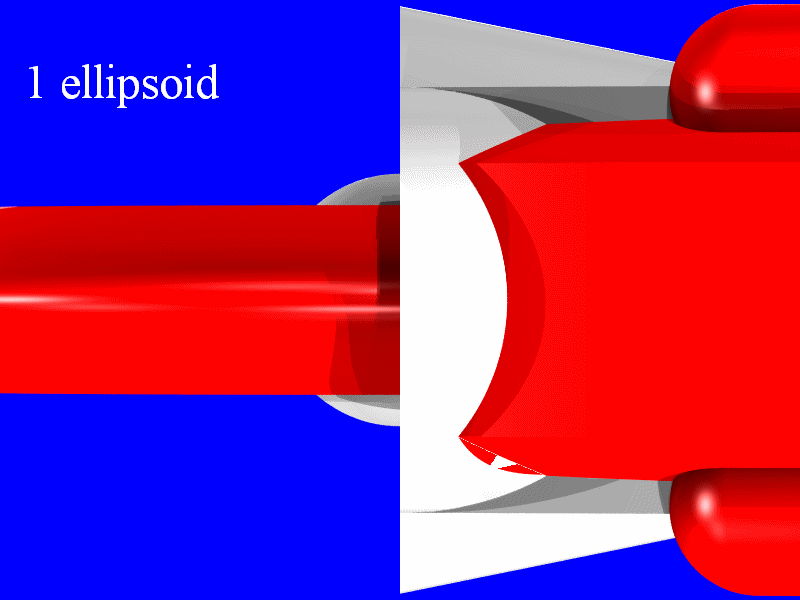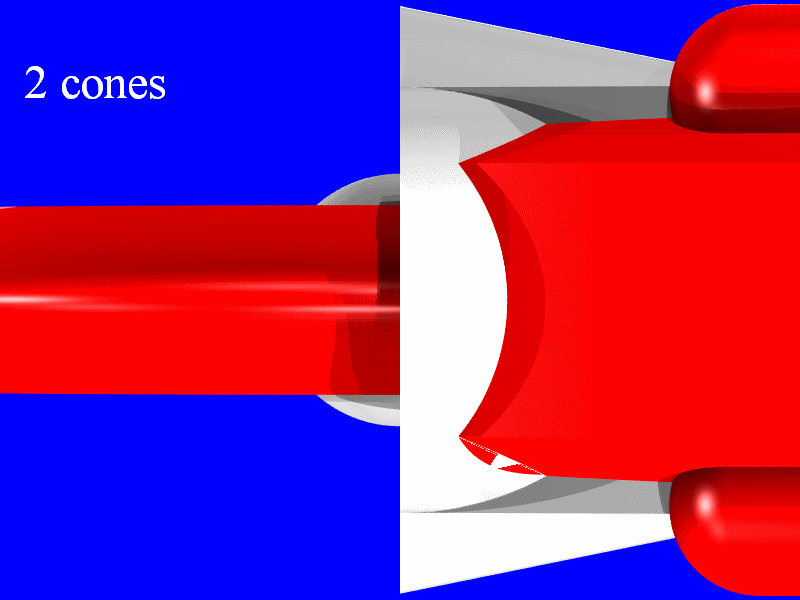
What do you think?
Carl

|
luggage
User

Posts: 0 | Re: MAGI's CSG Primitives?
on Tuesday, August, 15, 2006 5:13 AM
wwwmwww Wrote:| matthew180 Wrote:Absolutely! But every image we see, i.e. the construction sequence on the DVD, and the image with view-frame overlay, show those side pieces as three or so primitives... I think they could do it with one, as we have just seen. |
I agree. Maybe the reason they broke it up was to give them more pieces to play with for the crash effect. Maybe they wanted to restrict themselves to a simple subset of the primitives available to them just to aid in the computer generated look of the light cycle. It's also possible rendering times played a factor in their selection of primitives to use.
| matthew180 Wrote:Also, what about the third shape in the first row? Look at that surface, the curves are pretty complex and is either a mesh of some sort, or a lot of subtractions. Anyway, I found the images very enlightening. |
I think its either what POV-Ray would call a Isosurface Object or something similiar to what POV-Ray calls a Bicubic Patch Object.
I'm leaning toward the Isosurface as I tend to think of the Bicubic Patch as a type of 2D mesh. However the lines on the shape make me think of the control points used to define a Bicubic Patch so maybe they could define a function with the use of control points in their Isosurface generator.
Check out the torus like shape in the second row. It also has the extra lines and it appears the hole in the center has a square cross-section. If that's the case it almost has to be an Isosurface.
| matthew180 Wrote:As for the canopy taper, I think I'll try a torus as you have shown and see what kind of intersection I get with the fender. Thanks for the graphic. |
I'll do the same and see what I get. I was about 99% of the way there yesterday but just ran out of time.
Carl |
Hi all. I just wanted to add some remarks from a programmers point of view. Freeform surfaces (not meshes) is a relatively modern form of modelling, I think we can savely assume that MAGI did not use any kind of bicubic patches or the like. I am also quite sure MAGI have had no possibility to define polygons or lathes thereof - the biggest problem with meshes is the amount of data required to define a shape (plus numerous possibilities to define invalid shapes (.i.e not all points on a plane etc). That is quite contrary to the "simplicity" of their other shapes. I think they entered all of their animation data by hand, surely they were really keen on minimising the amount of data as much as possible.
However, it is trivially easy to define the most complex surfaces when having a general "implicit surfaces solver" at hand. This is what Carl described as beeing an "Isosurface" in povray.
An implicit surface defines a a surface as the zero contour of a function F(x,y,z) = 0. Imaging the "primitives" that could be described by general solver (sth. like F(x,y,z) = x^2+y^2+z^2-3 would be a sphere). Implicit surfaces also blend so well with CSG and are rather straighforward for mathematicians (from what I know most of the folks at MAGI were mathematicians).
There is only one problem: A general solver is quite slow 
Anyways, I think MAGI was more in stuff like this than in polygons.
Martin
|
luggage
User

Posts: 0 | Re: MAGI's CSG Primitives?
on Tuesday, August, 15, 2006 5:13 AM
btw: keep on the modelling 
Martinwhere to buy abortion pill ordering abortion pills to be shipped to house buy abortion pill online
|
|